Pythagoras

Pythagoras

Lat: 63.5°N, Long: 63.0°W, Diam: 142 km, Depth: 3.78 km, Rükl: 2, Upper Imbrian

Table of Contents

[#Pythagoras Pythagoras]

[#Pythagoras-Images Images]

[#Pythagoras-Maps Maps]

[#Pythagoras-Description Description]

[#Pythagoras-Description: Elger Description: Elger]

[#Pythagoras-Description: Wikipedia Description: Wikipedia]

[#Pythagoras-Additional Information Additional Information]

[#Pythagoras-Nomenclature Nomenclature]

[#Pythagoras-LPOD Articles LPOD Articles]

[#Pythagoras-Bibliography Bibliography]

LO-IV-176H

Images

LPOD Photo Gallery Lunar Orbiter Images SMART-1(mirror reversed)

• Kaguya HDTV 006_3_l shows Pythagoras viewed obliquely from the northwest with Babbage in the distance.

Maps

(LAC zone 10B1) USGS Digital Atlas PDF

Description

Description: Elger

(IAU Directions) PYTHAGORAS.--A noble walled-plain, 95 miles in diameter, which no one who observes it fails to lament is not nearer the centre of the disc, as it would then undoubtedly rank among the most imposing objects of its class. Even under all the disadvantages of position, it is by far the most striking formation in the neighbourhood. Its rampart rises, at one point on the N., to a height of nearly 17,000 feet above the floor, on which stands a magnificent central mountain, familiar to most observers.

Description: Wikipedia

Pythagoras

Additional Information

• Depth data from Kurt Fisher database
  • Westfall, 2000: 5.25 km
  • Viscardy, 1985: 4.8 km
  • Cherrington, 1969: 4.99 km
• Measures on LRO QuickMap give depth about 3.8 km, lower than I would expect!
• The sun angle in LO-IV-176H is too high to make a valid estimate of the crater depth, but the shadows cast by the slumped areas along the eastern wall indicate those blocks must be roughly 3,400 m tall, which is consistent with the higher values given for the crater rim in Kurt Fisher's database.

• Central peak heights
  • As measured by LTVT, the three central peaks in LO-IV-176H have heights, listing them from west to east of 1920 m, 1990 m, 2350 m, but only in the first case does the tip of the shadow fall on the crater floor. So the latter two are lower limits on the height of those peaks, and, in fact, misleading since Clementine images, taken with the sun to the south, show that the shape of the apparent triangle of shadow being cast by the right-most "peak" is really an artifact arising from the way the shadow falls on steeply sloping terrain, extended by a low ridge descending to the north. The main right-hand summit is at the bottom corner of the triangle. The shadows in Clementine LUB4662P.192 show that the tall center and right-most peaks rise 3.27 km and 3.18 km above the crater floor, with the low point between them descending to 2.82 km. - JimMosher JimMosher
  • Laser altimeter profile from Kaguya gives crater depths from 4.2-4.8 km, with central peak 3.0 km high. - tychocrater tychocrater Aug 19, 2008
• Measures on LRO QuickMap give central peak height about 2.8 km. This peak rises to the level of th3 surrounding terrain - very unusual! CAW

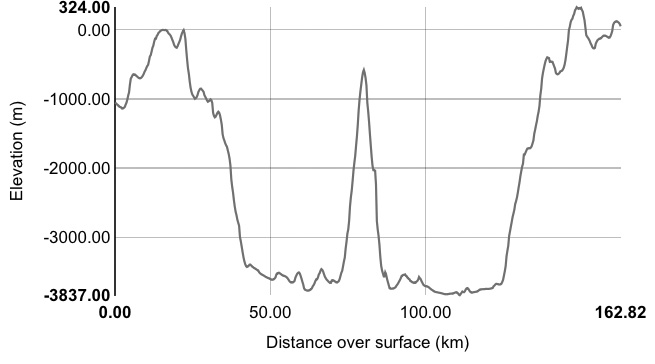
LRO QuickMap cross-section of Pythagoras

• • Sekiguchi, 1972:
    • 3.6 km "large, peaked mountain"
    • 1.8 km another mountain attached to the north of the one listed above
    • 0.1 km "mound . . . seen on the west slope of [the top mountain]"
    • "These three objects are generally regarded as one mountain block and named Alpha" - fatastronomer fatastronomer
• Central peak composition: A & GNTA1 (Tompkins & Pieters, 1999)
• Exterior impact melt deposits most extensive to NE, max of ~45 km beyond rim. Most extensive ejecta, rays and secondary craters to the S, with max wall slumping on S side of crater, and topographically lowest rim crest to NE (Hawke and Head, 1977).
• Stratigraphy changed to Upper Imbrian based on Galileo data and crater counts (McEwen et al, 1993)
• TSI = 35, CPI = 20, FI = 20; MI =75 Smith and Sanchez, 1973

Nomenclature

• Named for Pythagoras of Samos (between 580 and 572 BC–between 500 BC and 490 BC), an Ionian (Greek) philosopher and founder of the religious movement called Pythagoreanism. He is best known for the Pythagorean Theorem which bears his name. Known as "the father of numbers," Pythagoras made influential contributions to philosophy and religious teaching in the late 6th century BC.
• The name Pythagoras was first used by Michel Florent Van Langren (Langrenus) as Pythagorae. It is one of only three names from Van Langren's list which were anchored to their original locations throughout the entire history of selenography. The other two are Endymionis (IAU: Endymion) and Langreni (IAU: Langrenus).
• This name (as Pythagoras) has remained unchanged since its original use for this feature on Riccioli's map (Whitaker, p. 214).

LPOD Articles

What a Difference Two Weeks Makes.
Seeing Double: Pythagoras
Copernicus on the Limb
Textures (the very first bit of early sunlight on the central peak of the totally shadowed Pythagoras).

Bibliography

---

This page has been edited 1 times. The last modification was made by - tychocrater tychocrater on Jun 13, 2009 3:24 pm - afx3u2